Precision Penning Trap Mass Spectrometry

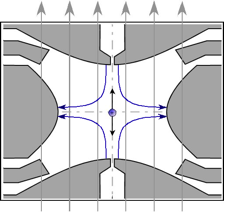
Cross-section of Penning trap electrodes showing the axial motion of a trapped ion. The direction of the uniform magnetic (vertical grey arrows), and of the electrostatic field (blue arrows) are also indicated.

Penning Trap Basics

A Penning trap consists of a set of electrodes located in a strong, uniform magnetic field. An ion of charge q and mass m, moving in a magnetic field B, experiences a Lorentz force qv x B, i.e, perpendicular to the magnetic field and the ion’s velocity v. Without the electrostatic potential this results in a simple circular motion, known as the cyclotron motion, in which the Lorentz force produces the centripetal acceleration. The cyclotron motion has an orbital frequency f_c = qB/2πm. This is the fundamental equation of Penning trap mass spectrometry. From this it follows that the ratio of the masses of two ions is the inverse of the ratio of their cyclotron frequencies, in the same magnetic field, for the same charge.

Because a uniform magnetic field cannot trap an ion in the direction parallel to the magnetic field an electrostatic potential is also applied using a set of electrodes surrounding the ion. Ideally, the electrodes produce a cylindrically symmetric potential varying quadratically along, and at right angles to the magnetic field. To trap a positive ion the endcaps of the trap are given a positive voltage with respect to the central ring electrode. Due to this electrostatic potential, the ion oscillates axially along the magnetic field direction. In the direction perpendicular to the magnetic field, the electric field is directed outwards, towards the ring. Without the magnetic field this would cause the ion to be accelerated from the trap center towards the ring. However, due to the qv xB Lorentz force, the result is a relatively slow, circular motion around the center of the trap, in which the Lorentz force and the outward electrostatic force nearly cancel. This is known as the magnetron motion. This motion causes the ion to be dynamically trapped in the plane perpendicular to the magnetic field. The ion still undergoes cyclotron motion. However, the center of the cyclotron motion, instead of being fixed, now follows the magnetron orbit. The frequency of the cyclotron motion is also reduced by the outward electrostatic force. In the ideal Penning trap, with a uniform magnetic field and a quadratic potential, the three motions are harmonic. In other words, the axial frequency f_z, the magnetron frequency f_m, and the modified cyclotron frequency f_ct are independent of the amplitudes (or radii) of the motions.

In fact, one can still use f_c = qB/2πm to determine mass ratios of ions in the presence of an electrostatic potential. However, now the ideal f_c is given in terms of the frequencies of the three measureable motions by the equation

f_c² = f_z² + f_ct² + f_m².

Remarkably, in the limit of small axial, magnetron and cyclotron amplitudes, this sums-of-squares expression for obtaining the ideal f_c holds true in the non-ideal Penning trap, in which, necessarily, the magnetic field deviates from uniformity, the electrostatic potential deviates from cylindrical symmetry, and the axis of the electrostatic potential can be misaligned from the axis of the magnetic field. This result, which is crucial to high precision measurement, is known as the Brown-Gabrielse theorem.